This article is the result of research carried out in the Federation " DAC - Crick & Croack ", managed by research specialdoc , manager of Biotek Team , who has cared for six months. All DAC confederates who attended a great big THANK YOU to specialdoc credit for everything flapping and thanks for letting me share the most fun, the data processing.
overconfidence (abbreviated as "OC"), I guess you already know what it is, however I take the manual, also considering the details communicated the message of the newsletter of August 2, 2010.
If you meet a team placed worse than you and your faith is strong or more, your players may underestimate their opponents and playing below their normal capabilities.
The risk of underestimating the opponent depends on the difference of points and position between the two teams, from your confidence and attitude of your team for the match. The greater the difference between the teams, the higher the confidence, the greater the risk. The only way to completely avoid the risk of underestimating the enemy is to play "game of the season."
If your team underestimate the opponent, the points of difference, the level of confidence, the attitude of the team and the camp factor, are the factors that determine the ' entity. Based on the result but you can recover in the interval between the two phases at a disadvantage if total recovery, 2 / 3 if in balance, 1 / 3 if leading by one goal.
overconfidence (abbreviated as "OC"), I guess you already know what it is, however I take the manual, also considering the details communicated the message of the newsletter of August 2, 2010.
If you meet a team placed worse than you and your faith is strong or more, your players may underestimate their opponents and playing below their normal capabilities.
The risk of underestimating the opponent depends on the difference of points and position between the two teams, from your confidence and attitude of your team for the match. The greater the difference between the teams, the higher the confidence, the greater the risk. The only way to completely avoid the risk of underestimating the enemy is to play "game of the season."
If your team underestimate the opponent, the points of difference, the level of confidence, the attitude of the team and the camp factor, are the factors that determine the ' entity. Based on the result but you can recover in the interval between the two phases at a disadvantage if total recovery, 2 / 3 if in balance, 1 / 3 if leading by one goal.
Note that the underestimation can only happen in the league, but not in the first three days.
So it seems quite clear: the probability that there is OC is linked to four variables:
- The Difference of Location
- The difference in points
- Level of Confidence
- L 'Attitude (Mots, Normal, Pic)
while the intensity the CB is linked to four variables:
- The difference in points
- Level of Confidence
- L 'Attitude (Mots, Norma, Pic)
- factor field
unlike 4 above is missing the difference of position and join the camp factor.
Research
DAC DAC was collected in a sample of 90 games. In three matches the attitude was "Game of the Season" (Mots) and there was no OC, by the book. The current sample is then of 87 matches. Let's see how varied the presence or absence of OC to change the 4 variables:
1) The difference Location
This value can vary from 2 to a maximum of 7 (where a team is first and 'Another eighth), these data:
Research
DAC DAC was collected in a sample of 90 games. In three matches the attitude was "Game of the Season" (Mots) and there was no OC, by the book. The current sample is then of 87 matches. Let's see how varied the presence or absence of OC to change the 4 variables:
1) The difference Location
This value can vary from 2 to a maximum of 7 (where a team is first and 'Another eighth), these data:

see in the first row in the case of difference of positions equal to 2 we have 8 cases in which there is no OC and no cases OC. With a difference of positions equal to 3 there are 5 cases with 2 cases of OC and OC, 2 out of 7 cases are 28.57%. And so on to grow. You see a difference in values \u200b\u200bof positions of 5, 6 or 7 of the OC seems to settle around the values \u200b\u200b45/50%.
If we represent in a chart we see how the odds of OC increases with increasing differences of opinion.
If we represent in a chart we see how the odds of OC increases with increasing differences of opinion.

2) The difference in points
In the sample this value varies from 0 to 30.
In the sample this value varies from 0 to 30.
 As expected, the likelihood of OC increases with points of difference, becoming almost a certainty for differences more than 20 points.
As expected, the likelihood of OC increases with points of difference, becoming almost a certainty for differences more than 20 points.  The rocking curve is normal in a sample so small, but the trend line is clear.
The rocking curve is normal in a sample so small, but the trend line is clear. 3) The Level of Confidence
Trust can take the values \u200b\u200bof 5 ("Strong"), 6 ("magnificent"), 7 ("slightly exaggerated") and 8 ("exaggerated").

for values \u200b\u200bof 5 there is no OC, with confidence equal to 6 28% of cases, with confidence equal to 7 about 47%, with confidence equal to just under 40 8 % (although this may be tainted by the few available data with confidence equal to 8). This is the
grafico della probabilità di OC al variare del livello di Fiducia:
grafico della probabilità di OC al variare del livello di Fiducia:

4) L' Atteggiamento (Mots, Normal, Pic)

Si passa dai 5 soli casi giocando normal (il 16%), al 46% dei casi giocando Pic.

Alla ricerca di una FORMULA
Ora quanto visto sopra ci dà un idea di massima di come vari la fiducia al variare delle singole variabili. Per stimare però quanto pesi una variabile rispetto all'altra occorre considerarle tutte insieme e fare un'analisi di regressione.
In sostanza si tratta to estimate the values \u200b\u200ba, b, c, d and e of the following
trying to get a formula of the probability of OC, the formula to be taken with forceps because of the narrowness of the sample.
The use of the term probability is a bit 'inappropriate given that the final value will be between 0 and 1, but may also be worth a bit' more or less.
you jump the technicalities and jump to the conclusion by saying that an estimate of this type did not give reliable results. Then I tried to think a bit 'and I realized that the variables "difference position" and "Difference points" are not mutually independent but are correlated (the higher the points difference, the greater the difference in position of the two teams in principle), unless they are completely rusted regression analysis of these things effectively requires mutual independence of variables.
I tried to exclude the "Difference Points", but the effectiveness of the estimate (for the R-square statistic) fell, while removing the "difference position" was obtained by a decent formula.
Moreover, the "difference position" is not even part of the 4 variables that regulate the intensity of the CB (see above quote from the manual), to exclude from the calculation of the probability that the CB does not seem senseless sacrifice (taking into account also said that as part of the information is still implicit in the difference in points).
placing the attitude of "1" if you play Normal and "2" if you play Pic, the formula of probability of OC seems to be:
In sostanza si tratta to estimate the values \u200b\u200ba, b, c, d and e of the following
Chance of OC = a * Diff.Posiz. Diff.Punti + + b * c * d * Attitude + Confidence + and
trying to get a formula of the probability of OC, the formula to be taken with forceps because of the narrowness of the sample.
The use of the term probability is a bit 'inappropriate given that the final value will be between 0 and 1, but may also be worth a bit' more or less.
you jump the technicalities and jump to the conclusion by saying that an estimate of this type did not give reliable results. Then I tried to think a bit 'and I realized that the variables "difference position" and "Difference points" are not mutually independent but are correlated (the higher the points difference, the greater the difference in position of the two teams in principle), unless they are completely rusted regression analysis of these things effectively requires mutual independence of variables.
I tried to exclude the "Difference Points", but the effectiveness of the estimate (for the R-square statistic) fell, while removing the "difference position" was obtained by a decent formula.
Moreover, the "difference position" is not even part of the 4 variables that regulate the intensity of the CB (see above quote from the manual), to exclude from the calculation of the probability that the CB does not seem senseless sacrifice (taking into account also said that as part of the information is still implicit in the difference in points).
placing the attitude of "1" if you play Normal and "2" if you play Pic, the formula of probability of OC seems to be:
Chance of Diff.Punti OC = 0.04 * + 0, 08 + 0.16 * Trust * Attitude - 1
also seems easy to remember, the decimal point are in fact 4 for the different points, 8 for 16 for the trust and the attitude.
How does this formula on the sample? Soon
said if it is less than 50% in 49 cases there is no OC and OC's in 7 cases (two of which are just above the limit, 52%)
if it is greater than 50% there is no OC in 8 cases (three of which are just below the limit, to 48%) and OC in 23 cases
if the formula is greater than the 70% do not have OC only 1 in 14 (7%)
if the formula is less than 30% OC is only in 3 cases out of 41 (still at 7%)
is in a chart 7 games in the very fortunate that there was no OC, although a formula value of more than 50% in the rest of the sample there was no OC, as required by the low value given by the formula.

Turning finally to the probability values \u200b\u200bcalculated for those parts of OC nel campione che invece hanno avuto OC

vedete che sono solo 4 i casi sfortunati di OC prevista inferiore al 40% e invece realizzatasi. 4 i valori compresi tra 40% e 50% (di cui 3 al 48%) e altri 23 con probabilità superiore al 50% in cui poi l'OC si è concretizzata.
Le Cause dell'OC
Il fatto che i decimali per la differenza di punti siano 4, quelli per la fiducia 8 e quelli per l'atteggiamento 16 non significa che queste variabili siano nel medesimo ordine di importanza, dato che variano su scale diverse (da 0 a 30 la differenza punti, da 5 a 8 la fiducia e da 1 a 2 l'atteggiamento). Portando all on the same scale that is replacing the values \u200b\u200bof the confidence value (confidence-5) * 10 and 0 if the attitude and 30 if Pic Normal, the formula becomes:
points short, the difference seems to weigh more than 5 times the value of Trust and 8 times the value of attitude.
Then - and this is important - if we find that they beat teams in OC, is not the pic itself to be the cause of overconfidence, but the fact that the pic is done by teams that have a lot of points advantage on the opponent.
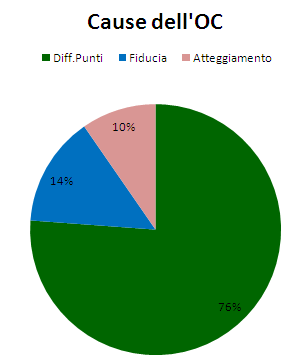
Overall, the OC depends for more than 75% of the difference points to the 14% level of confidence and 10% attitude.
I put in a pie chart that is perhaps most impressed:
Chance of OC * = 00:04 Diff.Punti + 0.0075 * Trust (scaled) + 0.005 * Attitude (rescaled) - 0.45
points short, the difference seems to weigh more than 5 times the value of Trust and 8 times the value of attitude.
Then - and this is important - if we find that they beat teams in OC, is not the pic itself to be the cause of overconfidence, but the fact that the pic is done by teams that have a lot of points advantage on the opponent.
Overall, the OC depends for more than 75% of the difference points to the 14% level of confidence and 10% attitude.
I put in a pie chart that is perhaps most impressed:
APPENDIX
This is the standard in detail:



PS. take a look at ' CONTENTS of the blog, there are several items that may be of interest.
Andreace (team in Hattrick ID 1730726)
Andreace (team in Hattrick ID 1730726)
This work is licensed by Andreace under a Creative Commons Attribution-Noncommercial 3.0 Unported License . Ie, this work may be freely copied, distributed or modified without the express permission of the author, provided that the author is clearly stated and the publication is not for commercial purposes.
0 comments:
Post a Comment